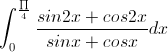Giải bất phương trình: \({\log _{0,5}}\left[ {{{\log }_3}\dfrac{{{x^2} + x + 1}}{{x + 1}}} \right] \ge 0\)
Câu hỏi
Nhận biếtGiải bất phương trình: \({\log _{0,5}}\left[ {{{\log }_3}\dfrac{{{x^2} + x + 1}}{{x + 1}}} \right] \ge 0\)
Đáp án đúng: D
Lời giải của Luyện Tập 365
\(\begin{array}{l}
{\log _{0,5}}\left[ {{{\log }_3}\dfrac{{{x^2} + x + 1}}{{x + 1}}} \right] \ge 0 \Leftrightarrow \left\{ \begin{array}{l}
\dfrac{{{x^2} + x + 1}}{{x + 1}} > 0\\
0 < {\log _3}\dfrac{{{x^2} + x + 1}}{{x + 1}} \le 0,{5^0}
\end{array} \right.\\
\Leftrightarrow \left\{ \begin{array}{l}
x + 1 > 0\\
1 < \dfrac{{{x^2} + x + 1}}{{x + 1}} \le 3
\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}
x > - 1\\
1 < \dfrac{{{x^2} + x + 1}}{{x + 1}}\\
\dfrac{{{x^2} + x + 1}}{{x + 1}} \le 3
\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}
x > - 1\\
\dfrac{{{x^2} + x + 1 - x - 1}}{{x + 1}} > 0\\
\dfrac{{{x^2} + x + 1 - 3x - 3}}{{x + 1}} \le 0
\end{array} \right.\\
\Leftrightarrow \left\{ \begin{array}{l}
x > - 1\\
\dfrac{{{x^2}}}{{x + 1}} > 0\\
\dfrac{{{x^2} - 2x - 2}}{{x + 1}} \le 0
\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}
x > - 1\\
x \ne 0\\
{x^2} - 2x - 2 \le 0
\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}
x > - 1\\
x \ne 0\\
1 - \sqrt 3 \le x \le 1 + \sqrt 3
\end{array} \right.
\end{array}\)
Vậy tập nghiệm của bất phương trình là \(\left[ {1 - \sqrt 3 ;1 + \sqrt 3 } \right]\backslash \left\{ 0 \right\}\)
\(\begin{array}{l}
{\log _{0,5}}\left[ {{{\log }_3}\dfrac{{{x^2} + x + 1}}{{x + 1}}} \right] \ge 0 \Leftrightarrow \left\{ \begin{array}{l}
\dfrac{{{x^2} + x + 1}}{{x + 1}} > 0\\
0 < {\log _3}\dfrac{{{x^2} + x + 1}}{{x + 1}} \le 0,{5^0}
\end{array} \right.\\
\Leftrightarrow \left\{ \begin{array}{l}
x + 1 > 0\\
1 < \dfrac{{{x^2} + x + 1}}{{x + 1}} \le 3
\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}
x > - 1\\
1 < \dfrac{{{x^2} + x + 1}}{{x + 1}}\\
\dfrac{{{x^2} + x + 1}}{{x + 1}} \le 3
\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}
x > - 1\\
\dfrac{{{x^2} + x + 1 - x - 1}}{{x + 1}} > 0\\
\dfrac{{{x^2} + x + 1 - 3x - 3}}{{x + 1}} \le 0
\end{array} \right.\\
\Leftrightarrow \left\{ \begin{array}{l}
x > - 1\\
\dfrac{{{x^2}}}{{x + 1}} > 0\\
\dfrac{{{x^2} - 2x - 2}}{{x + 1}} \le 0
\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}
x > - 1\\
x \ne 0\\
{x^2} - 2x - 2 \le 0
\end{array} \right. \Leftrightarrow \left\{ \begin{array}{l}
x > - 1\\
x \ne 0\\
1 - \sqrt 3 \le x \le 1 + \sqrt 3
\end{array} \right.
\end{array}\)
Vậy tập nghiệm của bất phương trình là \(\left[ {1 - \sqrt 3 ;1 + \sqrt 3 } \right]\backslash \left\{ 0 \right\}\)
Câu hỏi liên quan
-
Giải phương trình sin2x.(tan x - 1) = 3 sin x.(cos x + sin x) - 3.
-
Giải phương trình (1-
).![\sqrt[3]{2-x}](https://luyentap365.com/wp-content/picture/learning/exam/2013/1031/75_220_2.gif)
= x. -
Trong mặt phẳng với hệ trục Oxy, cho hình thoi ABCD biết phương trình của một đường chéo là 3x+y-7=0, điểm B(0;-3), diện tích hình thoi bằng 20. Tìm tọa độ các đỉnh còn lại của hình thoi.
-
Trong mặt phẳng với hệ trục Oxy, cho hai đường thẳng ∆1: 3x+y+5=0, ∆2: x-2y-3=0 và đường tròn (C):
+
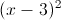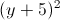=25. Tìm điểm M thuộc (C), điểm N thuộc đường thẳng ∆1, sao cho M và N đối xứng qua ∆2. -
Cho các số thực x,y thỏa mãn x
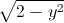+ y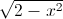
= 2 Tìm giá trị lớn nhất của biểu thức P=
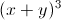-12(x-1).(y-1)+√xy. -
Trong không gian với hệ trục tọa độ Oxyz, cho mặt phẳng (P) và đường thẳng d lần lượt có phương trình (P): 2x-y-2z=0, d:
=
=
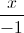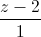Viết phương trình mặt cầu (S) có tâm thuộc đường thẳng (d), cách mặt phẳng (P) một khoảng bằng 3 và cắt mặt phẳng (P) một khoảng bằng 3 và cắt mặt phẳng (P) theo giao tuyến là đường tròn có bán kính bằng 4. -
Trong không gian với hệ trục tọa độ Oxyz, viết phương trình đường thẳng ∆ nằm trong mặt phẳng (P): x+y-z+1=0, cắt các đường thẳng 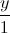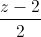d:
=
=
, d':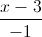
=
=
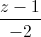và tạo với đường thẳng d một góc 
. -
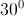Cho các số thực x, y, z không âm thỏa mãn điều kiện x3 + y3 + z3= 2 + 3xyz. Tìm giá trị nhỏ nhất của biểu thức P = x2 + 2y2 + 3z2.
-
Tính tích phân I =
-
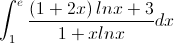
Tính tích phân I=
