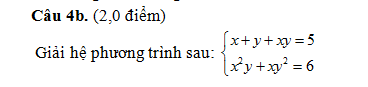
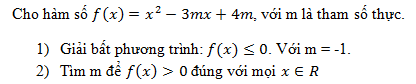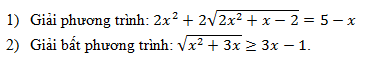Giải hệ phương trình:
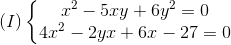

Câu hỏi
Nhận biếtGiải hệ phương trình:
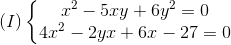
A.
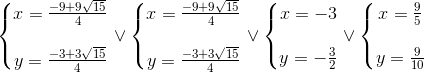
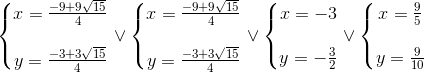
B.
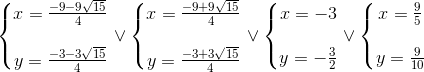
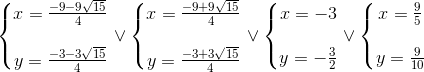
C.
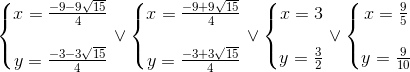
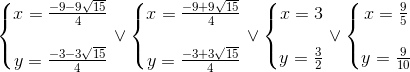
D.
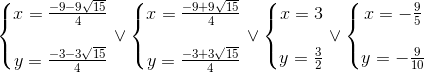
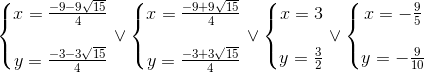
Đáp án đúng: B
Lời giải của Luyện Tập 365
Xem (1) < => x2 – (5y).x + 6y2 = 0 ( PT bậc 2 theo x )
=> x = 3y hoặc x = 2y lần lượt thế vào phương trình (2)
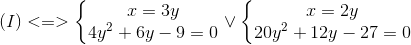
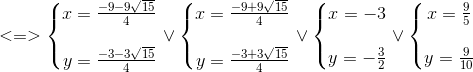
Vậy Hệ có 4 nghiệm.
Xem (1) < => x2 – (5y).x + 6y2 = 0 ( PT bậc 2 theo x )
=> x = 3y hoặc x = 2y lần lượt thế vào phương trình (2)
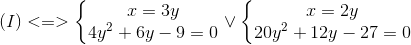
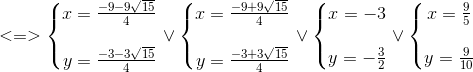
Vậy Hệ có 4 nghiệm.
Câu hỏi liên quan
-

Phần cơ bản
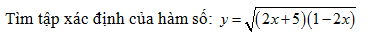

-

-

-

BAN CƠ BẢN

-

Cho góc
 thỏa mãn
thỏa mãn 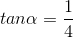 .. Tính các giá trị lượng giác còn lại của
.. Tính các giá trị lượng giác còn lại của 
-

Cho a, b, c, d là các số thực dương. Chứng minh rằng:
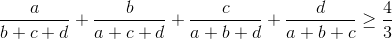
-

Tìm tập xác định của các hàm số sau:
a)
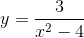
b)
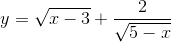
c)
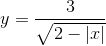
-

Giải và biện luận phương trình sau theo tham số m
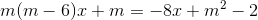
-

BAN NÂNG CAO
-

Phần nâng cao